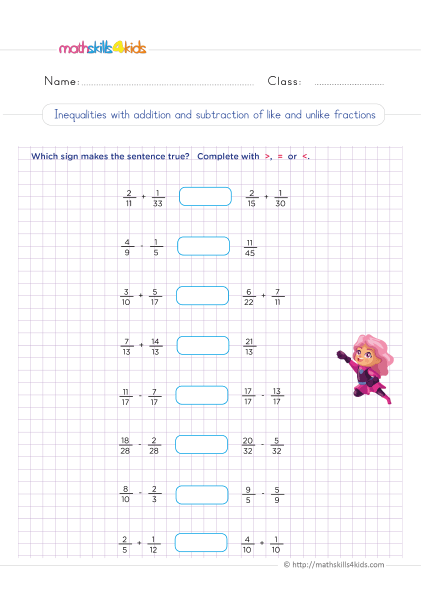
Adding and subtracting fractions worksheets for Grade 5
Hi there! Welcome to this exciting math article about fractions, addition, and subtraction. Here, we’ll share with you free adding and subtracting fractions worksheets for Grade 5 with fun teaching facts methods that will blow your mind and capture your children’s interest and love for more math activities.
-
Mastering fraction addition and subtraction: A step-by-step approach for 5th Graders
Are your 5th graders struggling with fraction addition and subtraction? Fear not because Mathskills4kids.com has the ultimate solution for you! In this article, we will take you through a step-by-step approach for 5th graders to quickly master fractions addition and subtraction. We understand that these concepts can be daunting for young learners, but with our expert guidance, your students will gain the confidence and skills to conquer fractions quickly.
We cover everything from understanding the basics of common denominators to simplifying fractions and finding the least common multiple. Our approach is designed to make learning fractions fun and interactive, with plenty of examples, practice exercises, and real-world applications to keep your students engaged.
So, whether you're a teacher looking for a resource to enhance your classroom instruction or a parent seeking to support your child's learning at home, this guide is your ticket to mastering fraction addition and subtraction. Let's dive in and unlock the world of fractions together!
-
BROWSE THE WEBSITE
-
DOWNLOAD FREE WORKSHEETS
-
-
5th GRADE MATH TOPICS
- Number sense
- Addition and subtraction
- Multiplication
- Division
- Exponents
- Number theory
- Decimals
- Add & subtract decimals
- Multiply decimals
- Divide decimals
- Fractions & mixed numbers
- Add & subtract fractions
- Multiply fractions
- Divide fractions
- Mixed operations
- Problems solving
- Ratios and rates
- Percentages
- Money Math
- Number sequences
- Coordinate graph
- Variable expressions
- Data and Graphs
- Probability and statistics
- Telling time
- Unit of measurements
- 2D figures
- Triangles & quadrilaterals
- Symmetry & transformations
- 3D figures
- Geometric measurements
-
-
Adding and subtracting fractions using a number line
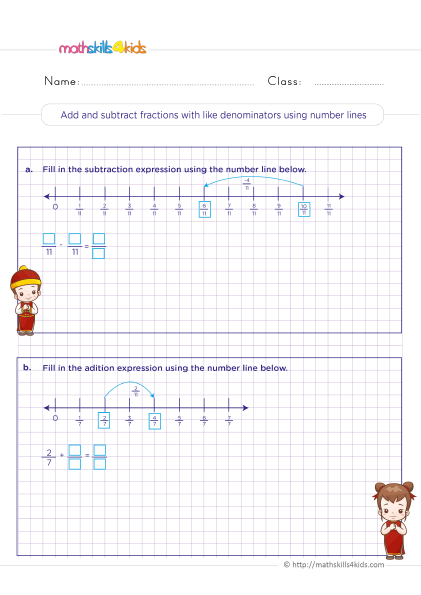 Print it...
Print it...
-
Adding and subtracting mixed numbers with unlike denominators
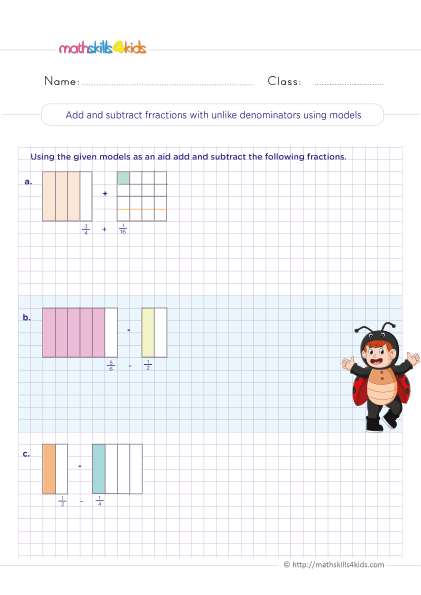 Print it...
Print it...
-
Adding up to 4 fractions with denominators of 10 and 100
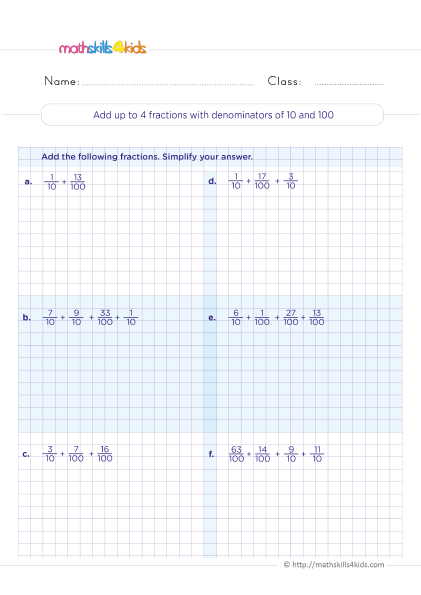 Print it...
Print it...
-
Estimating sums and differences of fractions using benchmarks
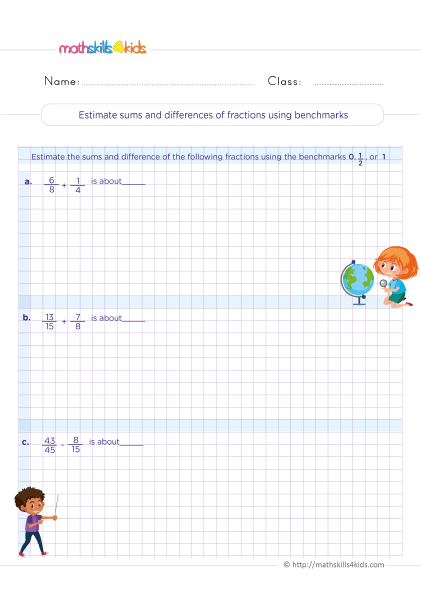 Print it...
Print it...
-
Add and subtract fractions with unlike denominators word problems
 Print it...
Print it...
-
Adding and subtracting 3 or more fractions word problems
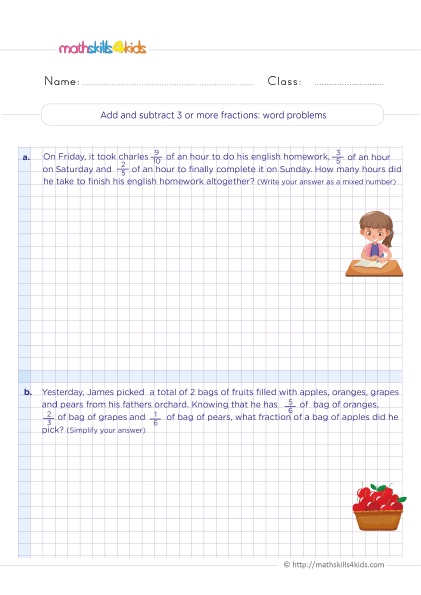 Print it...
Print it...
-
Adding and subtracting fractions using a number line
-
Buying is supporting us!
Buy Now...
-
-
Understanding the basics of fractions
Fractions are a fundamental concept in mathematics, representing a part of a whole. They consist of two numbers, a numerator, and a denominator, separated by a line. The numerator represents the number of parts we have, while the denominator represents the total number of equal parts that make up a whole. For example, in the fraction 3/4, 3 is the numerator, and 4 is the denominator.
Understanding the basics of fractions is crucial before diving into fraction addition and subtraction. Students should have a solid grasp of what fractions represent and how to read and write them. They should also understand the relationship between the numerator and denominator and how to compare fractions using greater than or less than symbols.
Once these foundational skills are in place, students will be ready to move on to adding and subtracting fractions.
-
Adding fractions with like denominators
When adding fractions with like denominators, the process is relatively straightforward. Add the numerators together while keeping the denominator the same. For example, if we want to add 1/4 and 2/4, we add the numerators, which give us 3, and keep the denominator as 4. Therefore, the sum of 1/4 and 2/4 is 3/4.
Providing students with plenty of examples and practice exercises is essential to reinforce this concept. Use visual aids such as fraction bars or circles to help students visualize the addition process.
Also, please encourage them to think about fractions in real-world contexts, such as dividing a pizza among friends or sharing a candy bar. By making the learning experience relatable and engaging, students will develop a deeper understanding of fraction addition.
-
Subtracting fractions with like denominators
Subtracting fractions with like denominators follows a similar process to adding fractions. The main difference is that instead of adding the numerators, we subtract them. The denominator remains the same. For example, if we want to subtract 2/5 from 3/5, we subtract 2 from 3, which gives us 1. The denominator remains 5, so the difference is 1/5.
To help students grasp subtracting fractions, provide them with concrete examples and practical applications. Please encourage them to think about situations where they need to subtract a part from a whole, such as eating a portion of a pie or giving away a fraction of their toys. By connecting the abstract concept of fraction subtraction to real-life scenarios, students will develop a deeper understanding and appreciation for fractions.
-
Adding fractions with unlike denominators
Adding fractions with unlike denominators requires an additional step: finding the least common denominator (LCD). The LCD is the smallest multiple that both denominators can divide evenly into. Once we have determined the LCD, we can convert both fractions to have the same denominator before adding them together.
To find the LCD, students should identify the factors of each denominator and choose the smallest common multiple. For example, if we want to add 1/3 and 1/5, we can follow the steps below:
- The factors of 3 are 1, 3, and the factors of 5 are 1, 5.
- The smallest common multiple is 15.
- To convert 1/3 to have a denominator of 15, we multiply the numerator and denominator by 5, resulting in 5/15.
- Similarly, to convert 1/5 to have a denominator of 15, we multiply the numerator and denominator by 3, resulting in 3/15.
- Finally, we can add 5/15 and 3/15 together to get 8/15.
It's essential to guide students through finding the LCD and converting fractions to like denominators. Please provide ample practice exercises and encourage them to check their answers by simplifying the fractions after addition. This will help reinforce their understanding of fraction addition with unlike denominators.
-
Subtracting fractions with unlike denominators
Subtracting fractions with unlike denominators follows the same process as adding fractions with unlike denominators. The only difference is that we subtract the numerators instead of adding them. Finding the LCD and converting the fractions to like denominators is still crucial before performing the subtraction.
For example, if we want to subtract 3/7 from 4/9,
- We first find the LCD, which is 63.
- We then convert both fractions to have a denominator of 63.
- To convert 3/7, multiply the numerator and denominator by 9, resulting in 27/63. To convert 4/9, we multiply the numerator and denominator by 7, resulting in 28/63.
- Finally, we subtract 27/63 from 28/63 to get 1/63.
To ensure students grasp the concept of subtracting fractions with unlike denominators, provide them with various practice exercises. Please encourage them to simplify their answers and check their work. Practicing regularly will make students more confident in subtracting fractions with unlike denominators.
-
Simplifying fractions after addition and subtraction
Simplifying fractions after addition and subtraction is another essential part of solving fractions procedure. Simplifying a fraction means reducing it to its lowest terms by dividing the numerator and denominator by their greatest common divisor. This step is crucial to ensure that fractions are expressed in their simplest form.
To simplify a fraction, students should identify the greatest common divisor (GCD) of the numerator and denominator. They can do this by finding the factors of both numbers and determining the largest common factor. For example;
If we have the fraction 4/8, the factors of 4 are 1, 2, and 4, and the factors of 8 are 1, 2, 4, and 8. The largest common factor is 4. Dividing the numerator and denominator by 4 gives us 1/2, which is the simplified form of 4/8.
Reinforce the importance of simplifying fractions by providing students with practice exercises and real-world examples. Please encourage them to think about situations where simplifying fractions is necessary, such as dividing a cake into equal parts or sharing a set of objects among friends. By practicing simplification, students will better understand fractions and how to express them in their simplest form.
-
Common mistakes to avoid in fraction addition and subtraction
Even with a step-by-step approach, students may still make common mistakes when adding and subtracting fractions. It's essential to highlight these mistakes and explain why they are incorrect. Some common mistakes to avoid in fraction addition and subtraction include:
- Forgetting to find the LCD when adding or subtracting fractions with unlike denominators.
- Adding or subtracting the denominators instead of the numerators.
- Incorrectly simplifying fractions after addition or subtraction.
- Forgetting to convert mixed numbers to improper fractions before performing addition or subtraction.
To help students avoid these mistakes, provide clear explanations and examples. Please encourage them to double-check their work and to simplify fractions whenever possible. By addressing these common mistakes early on, students will become more proficient in fraction addition and subtraction.
-
Practice exercises for adding and subtracting fractions worksheets for Grade 5
Practice makes perfect, and fraction addition and subtraction are no exception. Mathskills4kids.com will provide various practice exercises for adding and subtracting fractions worksheets for Grade 5 to reinforce kids’ solving skills and abilities.
- Start with simple problems and gradually increase the difficulty as students become more comfortable with the concepts.
- Include a mix of problems involving like and unlike denominators and problems that require simplification after addition or subtraction.
- Encourage students to show their work and explain their reasoning. This will help reinforce their understanding and develop their problem-solving and critical-thinking skills.
- Consider incorporating interactive activities like online games or manipulatives to make the practice sessions more engaging.
By providing students with opportunities to apply their knowledge in a fun and interactive way, they will develop a deeper understanding of fraction addition and subtraction.
Bonus: Resources for further learning and practice
Several resources are available to further enhance students' understanding and provide additional practice opportunities. Consider recommending the following:
- Online educational platforms: Websites like Khan Academy and IXL offer comprehensive lessons and interactive practice exercises on fraction addition and subtraction. Students can work at their own pace and receive immediate feedback on their progress:
- Math workbooks: Numerous math workbooks are designed for 5th-grade students. These workbooks typically include a variety of fraction-related exercises, including addition and subtraction.
- Math manipulatives: Manipulatives like fraction bars, fraction circles, or even everyday objects like paper clips or buttons can help students visualize and practice fraction addition and subtraction.
- Tutoring or after-school programs: Consider recommending tutoring or after-school programs specializing in math instruction. These programs can support and guide students needing extra help mastering fraction addition and subtraction.
Encourage students and parents to explore these resources and find the ones that best suit their learning preferences and needs. By providing various options, students can reinforce their learning and continue improving their fraction addition and subtraction skills.
-
-
Thank you for sharing the links of MathSkills4Kids.com with your loved ones. Your choice is greatly appreciated.
Conclusion
Mastering fraction addition and subtraction is an essential skill for 5th graders. By following a step-by-step approach and providing ample practice opportunities, students can build the confidence and skills to conquer fractions.
From understanding the basics of common denominators to simplifying fractions after addition and subtraction, mastering fraction operations may initially seem daunting. However, with dedication and practice, it becomes an achievable goal. Remember to highlight common mistakes to avoid and provide resources for further learning and practice.
With this article as your tool, teachers and parents can support their student's learning and help them unlock the world of fractions. So, let's embark on this exciting journey of adding and subtracting fractions worksheets for Grade 5 together, and watch as 5th graders become fraction superheroes!