Grade 5 algebraic expressions up to 2 variables worksheets
Learning what algebraic expressions are and how they can be solved is one of the most confusing parts of Grade 5 learning. That’s why we’ve put together this article, including thrilling Grade 5 algebraic expressions up to 2 variables worksheets to help tutors and parents explain this vital math topic to their 5th graders simply and effectively.
-
Solving algebraic expressions with ease: Grade 5 worksheets for 1 & 2 variable equations
Welcome to a world where algebraic expressions are no longer daunting for Grade 5 students! In this article, you’ll encounter special Grade 5 Worksheets for one and two Variable Equations from Mathskills4kids.com, designed to help you make solving algebraic expressions easy and fun for your students.
As children progress in their mathematical journey, it's crucial to strengthen their understanding of variables and equations. With our carefully crafted worksheets, students will gain confidence in tackling algebraic problems with a single variable and two variables. These worksheets are educational and engaging, ensuring that young learners stay motivated throughout the learning process.
Our expertly curated content perfectly balances simplicity and complexity, challenging students while providing step-by-step guidance. Whether it's simplifying expressions, solving for unknown values, or exploring real-world scenarios, our Grade 5 Algebraic Expressions up to 2 Variables Worksheets will equip students with the skills they need to excel in algebra.
Get ready to witness the transformation as algebraic expressions become second nature to your Grade 5 students!
-
BROWSE THE WEBSITE
-
DOWNLOAD FREE WORKSHEETS
-
-
5th GRADE MATH TOPICS
- Number sense
- Addition and subtraction
- Multiplication
- Division
- Exponents
- Number theory
- Decimals
- Add & subtract decimals
- Multiply decimals
- Divide decimals
- Fractions & mixed numbers
- Add & subtract fractions
- Multiply fractions
- Divide fractions
- Mixed operations
- Problems solving
- Ratios and rates
- Percentages
- Money Math
- Number sequences
- Coordinate graph
- Variable expressions
- Data and Graphs
- Probability and statistics
- Telling time
- Unit of measurements
- 2D figures
- Triangles & quadrilaterals
- Symmetry & transformations
- 3D figures
- Geometric measurements
-
-
Writing variable expressions word problems
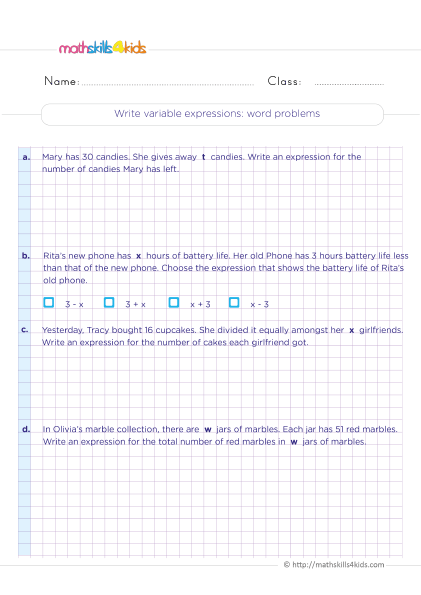 Print it...
Print it...
-
Evaluate variable expressions with whole numbers
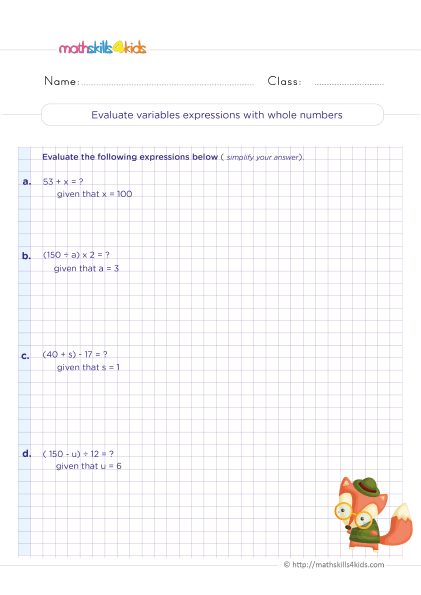 Print it...
Print it...
-
Writing variable equations word problems
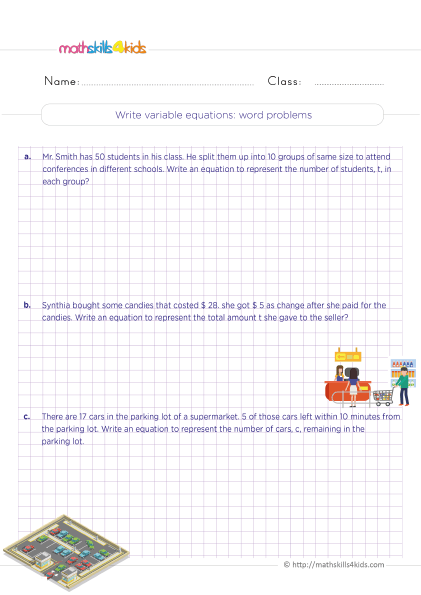 Print it...
Print it...
-
Evaluating multi-variables expressions practice
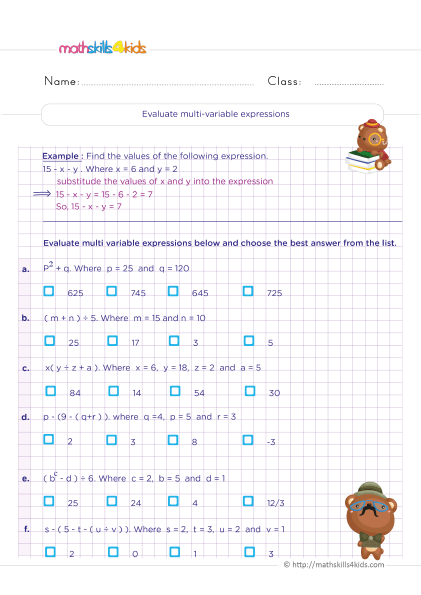 Print it...
Print it...
-
Complete solutions to 2-variable equations
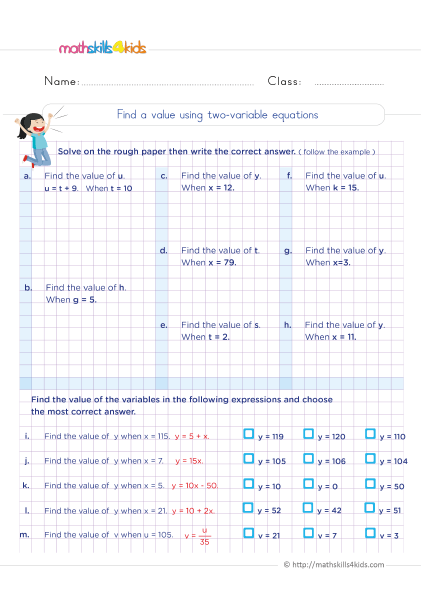 Print it...
Print it...
-
Completing solutions to two-variable equation practice
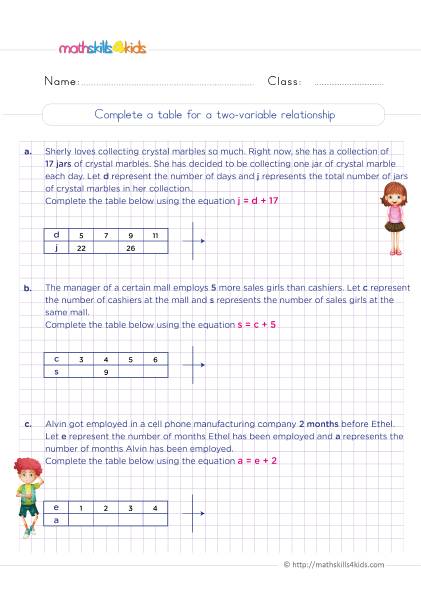 Print it...
Print it...
-
Writing variable expressions word problems
-
Buying is supporting us!
Buy Now...
-
-
What is the importance of mastering variable expressions in 5th grade?
Variable expressions are expressions that contain one or more letters that represent unknown numbers. For example, x + 3 is a variable expression that means "a number plus three." We can use variable expressions to write rules, patterns, formulas, and equations that describe different situations.
Mastering variable expressions in 5th grade is important because it helps students develop their algebraic thinking and reasoning skills. Algebra is the branch of mathematics that generalizes and abstracts numerical relationships. By learning how to use variables, you can:
- Represent and analyze patterns and functions
- Model and solve real-world problems
- Simplify and evaluate expressions
- Communicate and justify your mathematical ideas
In 5th grade, students will learn to write and evaluate variable expressions with up to two variables, such as 2x + y or 3a - b. They will also learn how to use the order of operations and the distributive property to simplify expressions. These skills will prepare 5th Grade students for more advanced topics in algebra, such as solving equations and inequalities, graphing linear functions, and working with exponents and radicals.
-
Understanding variables in algebraic expressions
Algebraic expressions often involve variables, which are symbols that represent unknown quantities. In Grade 5, students begin to encounter variables in their math lessons, laying the foundation for more complex algebraic concepts in the future. Understanding variables is essential as they allow us to generalize mathematical relationships and solve equations.
Variables can be represented by any letter, such as x, y, or z. These letters represent different values, which we can determine through algebraic manipulation. For example, if we have an equation 3x + 5 = 20, the variable x represents an unknown value. By solving the equation, we can find the value of x.
Variables are versatile and can be used in various algebraic expressions. Students will learn to identify variables, differentiate them from constants (fixed values), and manipulate them to solve equations.
-
Solving one-variable equations
One-variable equations involve a single unknown value represented by a variable. Solving these equations requires isolating the variable on one side of the equation to determine its value. Grade 5 students will learn different techniques to simplify and solve these one-variable equations.
A step-by-step guide to solving one-variable equations
To solve a one-variable equation, follow these steps:
- Simplify both sides of the equation by combining like terms and performing any necessary operations.
- Isolate the variable by moving constants to the other side of the equation.
- Perform inverse operations to eliminate any coefficients attached to the variable.
- Check your solution by substituting the value back into the original equation and verifying its correctness.
Let's consider an example equation: 2x + 3 = 9. To solve this equation, we can follow the steps mentioned above:
- Simplify both sides: 2x + 3 - 3 = 9 - 3, which gives us 2x = 6.
- Isolate the variable: We can do this by subtracting 3 from both sides, which gives us
2x = 6 - 3 or 2x = 3.
- Eliminate the coefficient: We can divide both sides by 2, resulting in x = 3/2 or x = 1.5.
- Check the solution: Substitute x = 1.5 back into the original equation: 2(1.5) + 3 = 9. Simplifying further, we get 3 + 3 = 9, which is true. Hence, the solution x = 1.5 is correct.
Practice worksheets for solving one-variable equations
To reinforce the understanding of one-variable equations, Mathskills4kids’ Grade 5 Worksheets provide ample practice opportunities. These worksheets are designed to gradually increase complexity, allowing students to develop their problem-solving skills at their own pace.
Each worksheet includes a variety of equations to solve, ensuring a comprehensive understanding of the concept. Through consistent practice, students will become more confident in solving one-variable equations and applying their mathematical knowledge.
-
Solving two-variable equations
As students progress in their mathematical journey, they will encounter equations with two variables. These equations involve two unknown values, and solving them requires finding values that satisfy the equation for both variables simultaneously. Solving two-variable equations introduces students to the concept of coordinate systems and graphing.
A step-by-step guide to solving two-variable equations
Solving two-variable equations follows a similar process to one-variable equations, with the addition of graphing techniques. Here's a step-by-step guide to solving two-variable equations:
- Simplify both sides of the equation by combining like terms and performing any necessary operations.
- Isolate one variable on one side of the equation.
- Substitute the value of the isolated variable into the other equation.
- Solve the resulting one-variable equation.
- Substitute the value found into either of the original equations to find the value of the second variable.
- Check the solution by substituting the values back into both equations and verifying their correctness.
Let's consider an example equation system:
2x + 3y = 12
x - y = 1
To solve this system of equations, we can follow the steps mentioned above:
- Simplify both sides of the equations: 2x + 3y = 12 and x - y = 1.
- Isolate one variable: Let's isolate x in the second equation by adding y to both sides, resulting in x = 1 + y.
- Substitute the value of x into the first equation: 2(1 + y) + 3y = 12. Simplifying further, we get 2 + 2y + 3y = 12, which gives us 5y = 10.
- Solve the resulting one-variable equation: By dividing both sides by 5, we find that y = 2.
- Substitute the value of y into either of the original equations: Let's use the second equation, x - y = 1. Substituting y = 2, we get x - 2 = 1, which gives us x = 3.
- Check the solution: Substitute x = 3 and y = 2 back into both equations. Both equations hold true, confirming the correctness of the solution.
Practice worksheets for solving two-variable equations
Mathskills4kids.com provide engaging practice worksheets for 5th graders to solve two-variable equations easily. These worksheets include various equation systems with two variables, allowing students to strengthen their problem-solving skills. By practicing on these worksheets, students will gain confidence in solving two-variable equations and interpreting the solutions in real-world scenarios.
-
Common mistakes to avoid when solving algebraic expressions
While solving algebraic expressions, students may encounter common mistakes that hinder their progress. It's essential to address these mistakes and provide guidance to avoid them.
- Incorrectly distributing operations. Students may forget to apply operations to all terms within parentheses or brackets when simplifying expressions. This mistake can lead to incorrect solutions and unnecessary confusion. Encourage students to double-check their work and ensure that operations are correctly distributed.
- Mixing up positive and negative signs. Algebraic expressions often involve positive and negative numbers, and students may accidentally switch signs or forget to include them altogether. Emphasize the importance of carefully maintaining the correct signs throughout the solving process to avoid errors.
- Unable to isolate variables due to improper application of inverse operations. Remind students to perform inverse operations on both sides of the equation to maintain equality and accurately isolate the variable.
-
10 variable equations word problems involving real-life situations with solutions
Now that students know why variable expressions are important let's practice using them to solve word problems. Word problems use words and numbers to describe a situation that a mathematical equation can model. To solve word problems, you need to:
- Read and understand the problem
- Identify the unknowns and assign variables to them
- Write an equation that relates the variables and the given information
- Solve the equation for the desired variable
- Check your solution and answer the question
Here are ten-word problems that involve variable expressions with up to two variables. Try to write the solutions to these problems as variable expressions, then check the solutions below.
- A rectangle has a length of l cm and a width of w cm. The perimeter of the rectangle is 36 cm. What are the possible values of l and w?
- A bag contains red and blue marbles. The number of red marbles is 3, more than twice the number of blue marbles. If there are 21 marbles, how many red marbles are there?
- A car rental company charges $25 per day plus $0.15 per mile for renting a car. How much will renting a car for 3 days cost if you drive x miles?
- A school club sells candy bars for $1 each. The club spent $50 to buy the candy bars and wants to make a profit of at least $100. How many candy bars must they sell?
- A triangle has a base of x cm and a height of y cm. The area of the triangle is 24 cm^2. What are the possible values of x and y?
- A plumber charges $40 for a service call plus $25 per hour for labor. How long did it take the plumber to fix a leak if the total bill was $165?
- A cookie recipe calls for x cups of flour and y cups of sugar. If you want to make half of the recipe, how much flour and sugar do you need?
- A train travels at a constant speed of x km/h for y hours. How far does the train travel in kilometers?
- A shirt costs $12 after a 20% discount. What was the original price of the shirt before the discount?
- A class has x boys and y girls. The ratio of boys to girls is 3:4. How many students are in the class?
Solutions:
Here are the solutions of the variable equations as variable expressions:
- l = 18 - w/2 and w = 36 - 2l
- r = 2b + 3 and b = (21 - r)/2
- C = 25*3 + 0.15x
- n = (50 + 100)/1
- x = 48/y and y = 48/x
- t = (165 - 40)/25
- f = x/2 and s = y/2
- d = xy
- p = 12/0.8
- x = 3y/4 and y = 4x/
Bonus: where to find more variable equations worksheets to boost 5th graders' math skills
If you're looking for more variable equations worksheets to boost your 5th graders' math skills, here are some of the best online resources offering free and printable Grade 5 algebraic expressions up to 2 variables worksheets.
You'll find a variety of exercises that will challenge your students and help them master the basics of algebra. Let's get started!
- One-Step Equations with Integers: This worksheet has 20 problems for students to solve equations with one variable and integer coefficients. The answer key is included. You can download it here: https://www.mathworksheets4kids.com/one-step-equation.php.
- Evaluating Expressions with x an y: This worksheet has 10 problems where students will evaluate expressions with one or two variables given their values. The answer key is included. You can download it here: https://www.k5learning.com/free-math-worksheets/fifth-grade-5/algebra/expressions-2-variables.
- Solving Equations with Distributive Property: This worksheet lets you create up to 15 problems where students have to solve equations with variables on both sides and apply the distributive property. You can choose the types of coefficients and whether they are positive or negative. You can create it here: https://www.math-aids.com/Algebra/Algebra_1/Distributive_Property/Solving_Equations.html.
-
-
Thank you for sharing the links of MathSkills4Kids.com with your loved ones. Your choice is greatly appreciated.
Conclusion
In conclusion, our Grade 5 Worksheets for one and two Variable Equations provide a comprehensive and engaging resource for students to master algebraic expressions.
By strengthening their understanding of variables and equations, students will gain confidence in solving one and two-variable equations. The step-by-step guides and practice worksheets ensure a thorough understanding of the concepts, allowing students to excel in algebra and apply their skills to real-world scenarios.
With Mathskills4kids Grade 5 Worksheets, algebraic expressions will no longer be a daunting task but a fascinating journey of discovery for Grade 5 students.
