Grade 6 Math Skills: Fun and educational percentages worksheets
-
Do you want to help your 6th-graders master the skill of working with percentages? Do you want to make math fun and engaging for them? Do you want to prepare them for real-world situations that involve percentages? If you answered yes to these questions, this article is for you!
In this article, you will find everything you need to help increase your Grade 6 student’s math skills in solving percentage problems. Also, you will discover a wide variety of fun and educational percentages worksheets designed by Mathskills4kids.com experts aimed to engage students in finding percentages of numbers and converting between fractions, decimals & percentages easily.
Fun and effective ways to practice percentages skills in Grade 6 Math
If you need fun and effective ways to practice percentage skills with your students in Grade 6 Math, look no further than this fantastic article.
In this article, we will explore the importance of percentages for 6th-graders, why percentages matter in everyday life, and how to teach and practice percentage skills using fun and educational worksheets. We will also provide some tips and resources for finding more activities and games to make learning percentages a breeze.
-
BROWSE THE WEBSITE
-
DOWNLOAD FREE WORKSHEETS
-
-
GRADE 6 MATH TOPICS
- Whole numbers
- Multiplication
- Division
- Exponents and square roots
- Number theory
- Decimals
- Add & subtract decimals
- Multiply & divide decimals
- Fractions & mixed numbers
- Add & subtract fractions
- Multiply fractions
- Divide fractions
- Integers
- Operations with integers
- Mixed operations
- Rational numbers
- Problems solving
- Ratio & proportions
- Percentages
- Measuring units
- Money math
- Consumer math
- Telling time
- Coordinate graph
- Algebraic expressions
- One step equations
- Solve & graph inequalities
- Two-step equations
- 2D Geometry
- Symmetry & transformation
- 3D Shapes
- Geometry measurement
- Data and Graphs
- Statistics
- Probability
-
-
Percentages with percent grids
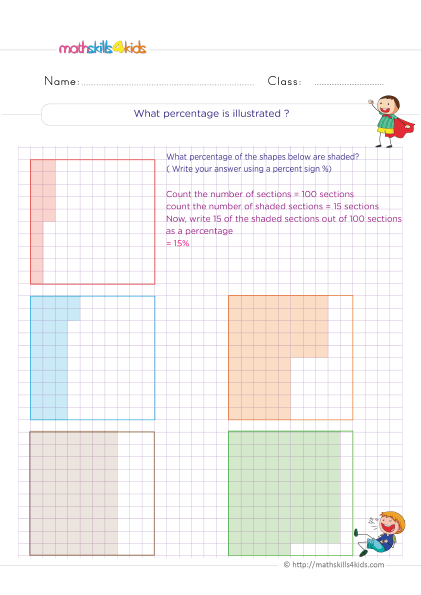 Print it...
Print it...
-
Convert between percents, fractions and decimals
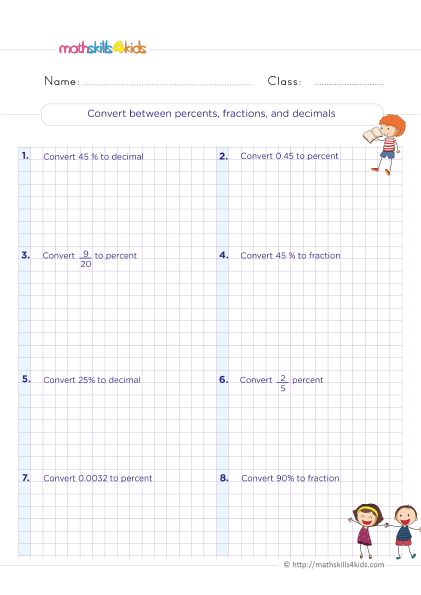 Print it...
Print it...
-
Comparing percents to each order and to fractions
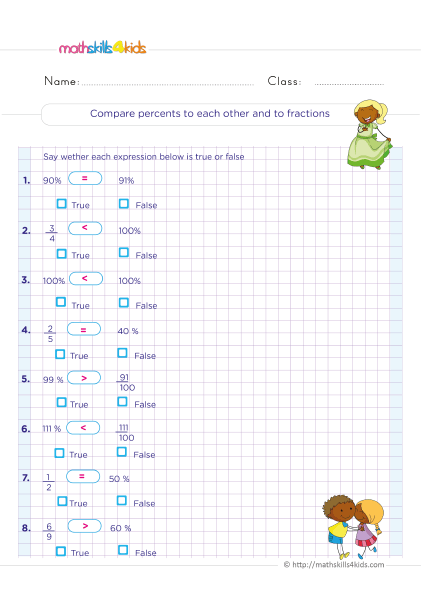 Print it...
Print it...
-
Money percentages practice
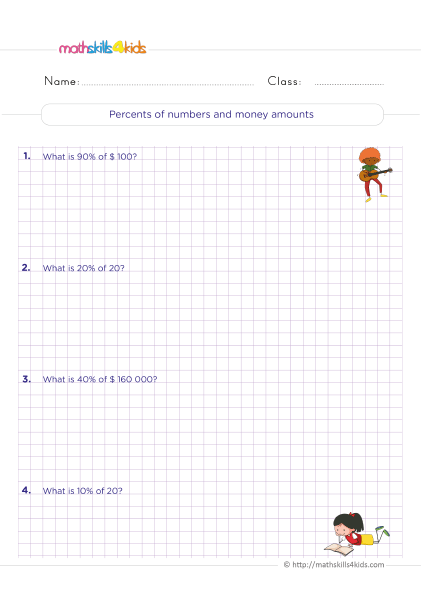 Print it...
Print it...
-
Fractions, decimals and percents
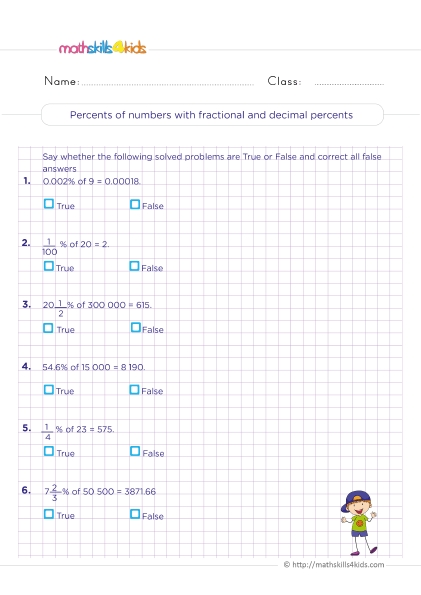 Print it...
Print it...
-
Finding what percent one number is of another word problems
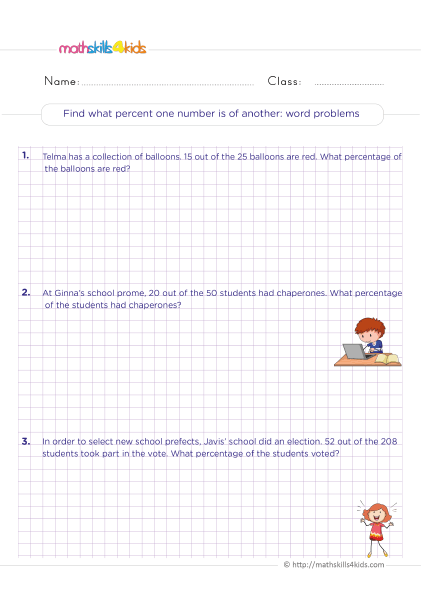 Print it...
Print it...
-
Percentages with percent grids
-
Buying is supporting us!
Buy Now...
-
-
Importance of percentage for 6th-graders
Before we start exploring the concept of percentage, let’s see why it is important to 6th graders.
Percentage is a concept introduced in the grade 6 math curriculum, and it is one of the essential skills that students need to master before moving on to higher-level math topics. Percentage is a way of expressing a part of a whole as a fraction of 100. For example, 50% means 50 out of 100, or half of something.
Percentage is closely related to fractions and decimals, which are also essential in grade 6 math. By learning how to convert fractions and decimals to percentages, and vice versa, students can develop a deeper understanding of the relationships between different forms of numbers. This will help them compare, order, and manipulate numbers more efficiently and accurately.
-
Why percentages matter in everyday life
Percentages are not only useful for math class but also for everyday life. We encounter percentages all the time, whether we realize it or not. Here are some examples of how percentages are used in real-world scenarios:
- Shopping: When we buy something on sale, we need to calculate how much we save by using the percentage off. For example, if a shirt is marked down from $20 to $15, we can find the percentage off by dividing the difference by the original price: ($20 - $15) / $20 = 0.25, or 25%.
We can also use percentages to compare prices and find the best deal. For example, if two stores offer different discounts on the same item, we can use percentages to see which gives us more value.
- Banking: When we save or borrow money, we need to understand how interest rates work. Interest rates are expressed as percentages, telling us how much we earn or pay over time.
For example, if we deposit $100 in a bank account that pays 5% interest per year, we can find how much money we have after one year by multiplying the principal by the interest rate: $100 x 0.05 = $5. This means we will have $105 in our account after one year.
Similarly, if we borrow $100 from a bank that charges 10% interest per year, we can find how much money we owe after one year by multiplying the principal by the interest rate: $100 x 0.1 = $10. This means we will have to pay back $110 after one year.
- Sports: We often use percentages to measure performance and outcomes when we watch or play sports. For example, we use shooting percentage in basketball to indicate how often a player makes a shot.
Shooting percentage is calculated by dividing the number of shots made by the number of shots attempted: shots made / shots attempted. For example, if a player makes 8 out of 10 shots, their shooting percentage is 8 / 10 = 0.8 or 80%.
We can also use percentages to predict the probability of winning or losing a game. For example, a team with a 60% chance of winning a game is expected to win 60 out of 100 times.
- Shopping: When we buy something on sale, we need to calculate how much we save by using the percentage off. For example, if a shirt is marked down from $20 to $15, we can find the percentage off by dividing the difference by the original price: ($20 - $15) / $20 = 0.25, or 25%.
-
Review: How to convert fractions and decimals to percentages
Before we move on to finding percentages of quantities, let's review how to convert fractions and decimals to percentages. This is a skill that your students learned in grade 5, but it is very useful for working with percentages in grade 6 and beyond.
To convert a fraction to a percentage, we need to divide the numerator (the top number) by the denominator (the bottom number) and then multiply by 100. For example;
- To convert 3/4 to a percentage, we do:
3 ÷ 4 = 0.75
0.75 x 100 = 75
So, 3/4 is equivalent to 75%.
- To convert a decimal to a percentage, multiply by 100. For example, to convert 0.6 to a percentage, you do:
0.6 x 100 = 60
So, 0.6 is equivalent to 60%.
We can also use these methods to convert percentages to fractions or decimals. To convert a percentage to a fraction, we will divide by 100 and simplify if possible. For example;
- To convert 80% to a fraction, you do:
80 ÷ 100 = 0.8
0.8 = 4/5
So, 80% is equivalent to 4/5.
- To convert a percentage to a decimal, we must divide by 100. For example, to convert 25% to a decimal, you do:
25 ÷ 100 = 0.25
So, 25% is equivalent to 0.25.
- To convert 3/4 to a percentage, we do:
-
Finding percentages of quantities using a calculator
Now that your 6th Grade students know how to convert fractions and decimals to percentages, they can use a calculator to find percentages of quantities. For example, if they want to find 20% of $50, they can do the following:
20 ÷ 100 = 0.2
0.2 x 50 = 10
So, 20% of $50 is $10.
They can also use this method to find the quantity when they know the percentage and the part. For example, if they know that 15% of a number is 9, they can do the following:
15 ÷ 100 = 0.15
9 ÷ 0.15 = 60
So, the number is 60.
-
Estimating percentages of shapes without a calculator
Sometimes, students may lack access to a calculator or want to estimate the percentage of a shape without doing any calculations. For example, if they see a pie chart or a bar graph, they may want to estimate the percentage of each category or section.
To do this, they will use their knowledge of fractions and decimals and compare them with common percentages. For example, if students see a shape divided into four equal parts, they can estimate that each part is about 25% since 1/4 is equivalent to 0.25 or 25%.
Similarly, if they see a shape divided into ten equal parts, you can estimate that each part is about 10% since 1/10 is equivalent to 0.1 or 10%.
Students can also use benchmarks such as half (50%), quarter (25%), third (33%), fifth (20%), and tenth (10%) to estimate percentages of shapes. For example, if they see a shape divided into three equal parts and one part is shaded, they can estimate that the shaded part is about 33% since it is one-third of the whole shape.
-
Comparing and ordering percentages using a number line
Another skill 6th graders will grasp in this concept is comparing and ordering percentages using a number line. A number line is a line that shows numbers in order from smallest to largest or vice versa. They can use it to compare and order fractions, decimals, and percentages by placing them on the same line.
For example, if we want to compare and order these percentages: 75%, 50%, 25%, and 10%, we can place them on a number line like this:
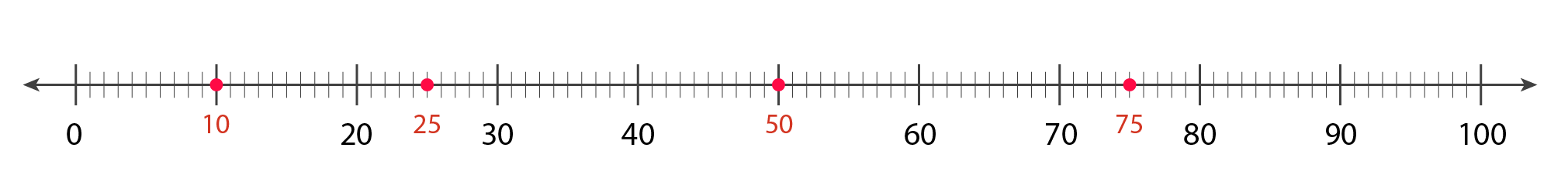
We can see that 10% is the smallest and 75% is the largest. We can also see that 25% is half of 50% and 50% is half of 100%. We can use these relationships to compare and order percentages more easily.
-
Solving word problems involving percentages
One of the most common applications of percentages is solving word problems. Word problems are problems that use words and numbers to describe a situation or a question. Students need to use their Grade 6 math skills and logic to find the answer.
To solve word problems involving percentages, ensure that your students follow these steps:
- Read the problem carefully and identify what is given and what is asked.
- Write an equation or a formula that relates the given information and the unknown quantity.
- Substitute the given values into the equation or formula and solve for the unknown quantity.
- Check their answer by plugging it back into the equation or formula and see if it makes sense.
For example, here is a word problem involving percentages:
A shirt costs $40 and is on sale for 20% off. What is the sale price of the shirt?
To solve this problem, we need to follow these steps:
- Read the problem carefully and identify what is given and what is asked.
Given: The original shirt price is $40, and the discount rate is 20%.
Asked: The sale price of the shirt.
- Write an equation or a formula that relates the given information and the unknown quantity.
One way to write an equation for this problem is:
- sale price = original price - discount amount
Another way to write an equation for this problem is:
- sale price = original price x (1 - discount rate)
Both equations are equivalent and will give the same answer.
- Substitute the given values into the equation or formula and solve for the unknown quantity.
Using the first equation, we get:
Sale price = $40 - discount amount
Discount amount = original price x discount rate
Discount amount = $40 x 0.2
Discount amount = $8
Sale price = $40 - $8
Sale price = $32
Using the second equation, we get:
Sale price = original price x (1 - discount rate)
Sale price = $40 x (1 - 0.2)
Sale price = $40 x 0.8
Sale price = $32
- Let’s check our answer by plugging it back into the equation or formula and see if it makes sense.
Using the first equation, we get:
$32 = $40 - discount amount
Discount amount = $40 - $32
Discount amount = $8
Discount rate = discount amount / original price
Discount rate = $8 / $40
Discount rate = 0.2 or 20%
Using the second equation, we get:
$32 = $40 x (1 - discount rate)
Discount rate = 1 - sale price / original price
Discount rate = 1 - $32 / $40
Discount rate = 1 - 0.8
Discount rate = 0.2 or 20%
Both equations give the same answer, and it makes sense that the sale price is less than the original price by 20%.
-
Applying percentages to real-world scenarios
One of the best ways to learn and practice percentages is to apply them to real-world scenarios. This helps us understand how percentages are used in everyday situations and how they can help us make decisions, compare options, and solve problems.
Here are some examples of real-world scenarios from Mathskills4kids’ fun and educational percentages worksheets:
- Shopping: When shopping, we may encounter discounts, sales taxes, and tips. These are all expressed as percentages of the original price. For example;
If a shirt is marked down 25%, we can find the new price by multiplying the original price by 0.75 (or 75%).
If the sales tax is 8%, we can find the total price by multiplying the new price by 1.08 (or 108%).
If we want to leave a 15% tip at a restaurant, we can find the amount by multiplying the total price by 0.15 (or 15%).
- Banking: We may encounter interest rates when we save or borrow money. These percentages indicate how much money we earn or pay over time. For example;
If we deposit $1000 in a savings account that pays 2% interest per year, we can find the amount after one year by multiplying $1000 by 1.02 (or 102%).
If we borrow $5000 from a bank that charges 5% interest per year, we can find the amount after one year by multiplying $5000 by 1.05 (or 105%).
- Sports: We may encounter statistics that measure performance or probability when we watch or play sports. These are often expressed as percentages or fractions. For example;
If a basketball player makes 8 out of 10 free throws, their free throw percentage is 80%.
If a soccer team has a 60% chance of winning a game, their probability of winning is 0.6 (or 60/100).
- Shopping: When shopping, we may encounter discounts, sales taxes, and tips. These are all expressed as percentages of the original price. For example;
Bonus: Where to find more resources and activities for teaching percentages
If you want to help your 6th Grade math learners learn more about percentages and practice their skills, there are many online resources and activities for teaching percentages you can download or print for them. Here are some of the best ones that we recommend:
- Math is Fun: Percentages (https://www.mathsisfun.com/percentage.html). This website comprehensively explains what percentages are, how to calculate them, and how to use them in different situations. It also has interactive exercises, quizzes, and games that students can try.
- Khan Academy: Percentages (https://www.khanacademy.org/math/cc-sixth-grade-math/x0267d782:cc-6th-rates-and-percentages/cc-6th-percentages/e/intro-to-percents). This website has video lessons, articles, and practice problems that cover everything 6th graders need to know about percentages. They can also track their progress and earn badges as they learn.
- Math Playground: Percent Games (https://www.mathplayground.com/bingo-find-a-percent-of-a-number.html). This website has fun and engaging games that help students practice finding, comparing, and applying percentages. They can also challenge themselves with word problems and puzzles that involve percentages.
- Math Goodies: Percent Worksheets (https://www.mathgoodies.com/worksheets/percent_wks). This website has printable worksheets that 6th graders can use to practice their percentage skills. You can choose from different difficulty levels and topics, such as finding the percent of a number, the whole given the part and the percent, and the percent change.
-
-
Thank you for sharing the links of MathSkills4Kids.com with your loved ones. Your choice is greatly appreciated.
Conclusion: How to Practice and Improve Your Percentage Skills
Percentages are an essential math skill in many aspects of our lives. They help us understand fractions, decimals, ratios, proportions, etc. They also help us compare and analyze data, make decisions, and solve problems.
To practice and improve percentage skills, 6th Grade students should:
- Review the basics of percentages, such as converting fractions and decimals to percentages, finding percentages of quantities using a calculator or mental math, and estimating percentages of shapes without a calculator.
- Practice comparing and ordering percentages using a number line, a table, or a graph. They should also be able to find equivalent percentages using fractions or decimals.
- Solve word problems involving percentages, such as finding the percent of increase or decrease, finding the original amount given the final amount and the percent change, and finding the discount or tax rate given the original and final prices.
- Apply percentages to real-world scenarios, such as calculating tips, interest rates, sales prices, grades, probabilities, etc. They should also be able to interpret and communicate their results using words, symbols, or diagrams.
- Use online resources and activities to learn more about percentages and practice their skills. They can watch videos, read articles, do exercises, play games, print worksheets, or do projects that involve percentages.
By following these steps, your 6th graders will become more confident and proficient in working with percentages. Remember that math is fun and useful when you understand it and apply it to your life. Happy learning!
