Grade 6 Probability Worksheets with Answers: Download Now
Hi, and welcome to the fantastic world of probability! This article is enriched with a vast collection of fun Grade 6 probability worksheets with answers that you can download now from Mathskills4kids.com and use at home or as classroom practice with your Grade 6 students.
In this article, your 6th graders will explore some fundamentals of probability and its application in the real world, like weather forecasting, population estimate, etc. Also, while exploring this article, you’ll discover the following:
-
- Flip a Coin, Roll a Die, Spin a Wheel: Practice with engaging 6th Grade Probability
- What is probability? Why is it important for 6th graders?
- Basic concepts: Outcomes, events, sample space, and probability models
- Calculating probability: Using fractions, decimals, and percentages
- Probability rules: Addition rule, multiplication rule, and complement rule
- Probability of compound events: Independent and dependent events, mutually exclusive and overlapping events
- Applications of probability: Games of chance, simulations, and experiments
- Bonus: Where can you find more Grade 6 probability practice resources? (web links with descriptions)
- Conclusion: Review of main ideas and tips for further practice
Flip a Coin, Roll a Die, and Spin a Wheel: Practice with engaging 6th Grade Probability.
Are your 6th graders ready to have some fun with probability? In this article, your 6th graders will enjoy practicing and learning about chance and uncertainty with engaging 6th Grade Probability. They will discover how to predict the outcomes of different situations, such as flipping a coin, rolling a die, or spinning a wheel.
So let's get started!
-
BROWSE THE WEBSITE
-
DOWNLOAD FREE WORKSHEETS
-
-
GRADE 6 MATH TOPICS
- Whole numbers
- Multiplication
- Division
- Exponents and square roots
- Number theory
- Decimals
- Add & subtract decimals
- Multiply & divide decimals
- Fractions & mixed numbers
- Add & subtract fractions
- Multiply fractions
- Divide fractions
- Integers
- Operations with integers
- Mixed operations
- Rational numbers
- Problems solving
- Ratio & proportions
- Percentages
- Measuring units
- Money math
- Consumer math
- Telling time
- Coordinate graph
- Algebraic expressions
- One step equations
- Solve & graph inequalities
- Two-step equations
- 2D Geometry
- Symmetry & transformation
- 3D Shapes
- Geometry measurement
- Data and Graphs
- Statistics
- Probability
-
-
Counting principles
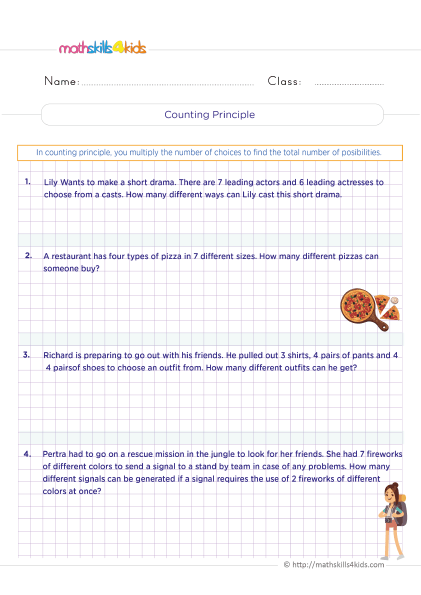 Print it...
Print it...
-
Find and calculate the probability of a single event
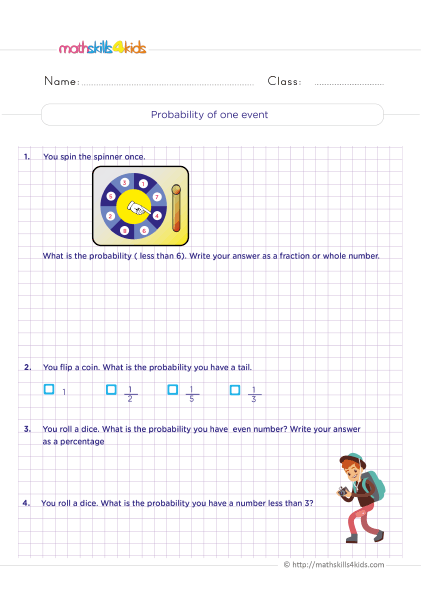 Print it...
Print it...
-
Make predictions
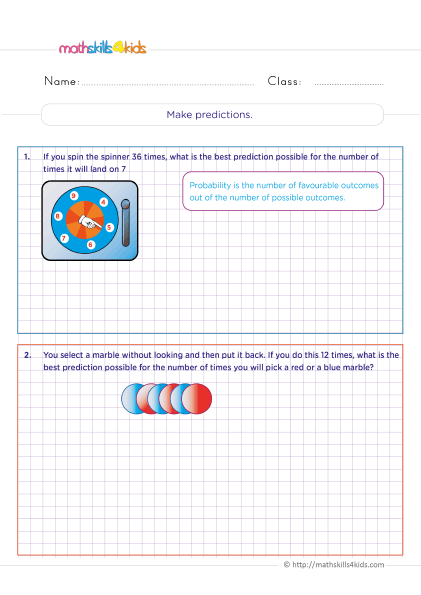 Print it...
Print it...
-
Compound probability: mutually exclusive vs overlapping answers
 Print it...
Print it...
-
How to determine if events are independent or dependent
 Print it...
Print it...
-
Simplifying factorial expressions
 Print it...
Print it...
-
Counting principles
-
Buying is supporting us!
Buy Now...
-
-
What is probability, and why is it important for 6th graders?
Probability is the measure of how likely something is to happen. For example, if you flip a fair coin, the probability of getting heads or tails is 50%. That means that out of 100 flips, you would expect to get heads about 50 times and tails about 50 times. Probability can be expressed as a fraction, a decimal, or a percentage. For example, 50% is the same as 1/2 or 0.5.
Probability is important for 6th graders because it helps them develop mathematical reasoning and critical thinking skills. It also helps them understand the concepts of randomness and variability, which are essential for data analysis and statistics. Probability can also be applied to real-life situations, such as making decisions, playing games, or conducting experiments.
-
Basic concepts: Outcomes, events, sample space, and probability models
Before we can calculate probability, we need to understand some basic concepts.
- An outcome is a possible result of an experiment or situation. For example, if you roll a die, an outcome could be 1, 2, 3, 4, 5, or 6.
- An event is a set of one or more outcomes we are interested in. For example, an event could be rolling an even number or a number greater than 4.
- A sample space is the set of all possible outcomes of an experiment or situation. For example, the sample space for rolling a die is {1, 2, 3, 4, 5, 6}.
- A probability model is a mathematical representation of an experiment or situation that shows the possible outcomes and their probabilities. For example, a probability model for flipping a coin could be a table like this:
Outcome
Probability
Heads
0.5
Tail
0.5
-
Calculating probability: Using fractions, decimals, and percentages
To calculate the probability of an event, we need to compare the number of favorable outcomes to the number of possible outcomes in the sample space. For example, if we want to find the probability of rolling an even number on a die, we need to count how many even numbers there are in the sample space {1, 2, 3, 4, 5, 6}. There are three even numbers: 2, 4, and 6. So, the number of favorable outcomes is 3. The number of possible outcomes is 6 because there are six sides on a die. So, the probability of rolling an even number is:
P(even) = favorable outcomes / possible outcomes
P(even) = 3 / 6
P(even) = 0.5
We can simplify this fraction by dividing the numerator and denominator by the same number. In this case, we can divide both by 3:
P(even) = (3 / 3) / (6 / 3)
P(even) = 1 / 2
We can also convert this fraction into a decimal by dividing the numerator by the denominator:
P(even) = 1 / 2
P(even) = 0.5
We can also convert this decimal into a percentage by multiplying it by 100 and adding a % sign:
P(even) = 0.5
P(even) = (0.5 x 100)%
P(even) = 50%
So, we can say that the probability of rolling an even number on a die is:
As a decimal = 0.5 or
As a fraction = 1/2 or
As a percentage = 50%.
-
Probability rules: Addition rule, multiplication rule, and complement rule
Now that your students know how to calculate the probability of simple events let's teach them some rules for finding the probability of more complex events. These probability rules are based on logic and common sense, making our lives easier when dealing with probability problems.
The first rule is the addition rule. It says that if you want to find the probability of one event or another event happening, you add their probabilities together. For example, if you flip a coin, the probability of getting heads is 1/2, and the probability of getting tails is also 1/2. So, the probability of getting heads or tails is 1/2 + 1/2 = 1. That makes sense, right? You are guaranteed to get either heads or tails when you flip a coin.
But wait, there is a catch. The addition rule only works when the events are mutually exclusive, which means they cannot happen simultaneously. For example, if you roll a die, the probability of getting a 1 is 1/6, and the probability of getting an even number is 3/6. But you cannot get a 1 and an even number at the same time, so they are mutually exclusive. In this case, the probability of getting a 1 or an even number is 1/6 + 3/6 = 4/6.
However, if the events are not mutually exclusive, which means they can happen simultaneously, then you have to subtract the probability of both events happening from the sum. For example, if you draw a card from a deck, the probability of getting a heart is 13/52, and the probability of getting a face card is 12/52. But you can simultaneously get a heart and face card, such as the King of Hearts. So, they are not mutually exclusive. In this case, the probability of getting a heart or a face card is 13/52 + 12/52 - 3/52 = 22/52. The 3/52 is the probability of getting both a heart and a face card, which we must subtract to avoid double-counting.
The second rule is the multiplication rule. It says that if you want to find the probability of one event and another event happening, you multiply their probabilities together. For example, if you flip two coins, the probability of getting heads on both coins is 1/2 x 1/2 = 1/4. That makes sense, too, right? You have to get heads on the first coin and then on the second to get heads on both.
But wait, there is another catch. The multiplication rule only works when the events are independent, meaning that one event does not affect the other. For example, if you roll two dice, the probability of getting a 6 on both dice is 1/6 x 1/6 = 1/36. That's because each die has no influence on what the other die shows.
However, if the events are not independent, which means that one event affects the other, then you have to adjust the probabilities accordingly. For example, if you draw two cards from a deck without replacing them, the probability of getting two hearts is not 13/52 x 13/52 = 169/2704. That's because after you draw the first card, there are only 51 cards left in the deck and only 12 hearts left. So, the probability of getting a heart on the second card is not 13/52 but 12/51. In this case, the probability of getting two hearts is 13/52 x 12/51 = 156/2652.
The third rule is the complement rule. It says that if you want to find the probability of an event not happening, you subtract its probability from 1. For example, if you flip a coin, the probability of not getting heads is 1 - 1/2 = 1/2. That's because there are only two possible outcomes: heads or tails. So if you don't get heads, you must get tails.
The complement rule is very useful when finding the probability of an event not happening is easier than the probability of an event happening. For example, if you roll a die, the probability of getting a prime number (2, 3, or 5) is difficult to calculate. But it is easy to find the probability of not getting a prime number (1, 4, or 6), which is just 3/6. So, by using the complement rule, we can find that the probability of getting a prime number is 1 - 3/6 = 3/6.
-
Applications of probability: Games of chance, simulations, and experiments
Apart from being a math concept, probability is a practical tool that can help us understand and predict real-world phenomena. One of the most common applications of probability is in games of chance, such as dice, cards, roulette, lottery, etc. These games are based on random outcomes that can be calculated using probability rules. For example, if you play the lottery, you can find the probability of winning the jackpot by multiplying the probabilities of matching each number on your ticket with the numbers drawn.
Another application of probability is in simulations, which are models that mimic real situations using random numbers. For example, if you want to estimate the average height of students in your school, you can use a simulation to randomly select a sample of students and measure their heights. Then, you can use the sample mean and standard deviation to estimate the population mean and standard deviation. Simulations can also be used to test hypotheses and compare different scenarios.
A third application of probability is in experiments, which are methods of collecting data by manipulating one or more variables and observing the effects. For example, if you want to test the effectiveness of a new drug, you can use an experiment to assign patients to either receive the drug randomly and a placebo or then measure their health outcomes. Then you can use statistical tests to determine if there is a significant difference between the two groups. Experiments can also be used to establish causal relationships and control for confounding factors.
Bonus: Where can you find more resources for Grade 6 probability practice?
If you want more resources for your 6th Grade probability practice, here are some helpful web links that you can check out:
- https://www.mathsisfun.com/data/probability.html: This website has clear explanations and examples of probability concepts, as well as interactive activities and quizzes.
- https://www.khanacademy.org/math/statistics-probability/probability-library: This website has video lessons, exercises on probability topics, articles, and tips.
- http://www.math-play.com/Probability.html: This website has fun and educational games that test students’ knowledge of probability.
- https://www.mathworksheets4kids.com/probability.php: This website has printable worksheets and answer keys on probability problems.
-
Thank you for sharing the links of MathSkills4Kids.com with your loved ones. Your choice is greatly appreciated.
Conclusion
We hope you have enjoyed reading this article about Mathskills4kids’ Grade 6 probability worksheets with answers and that your 6th graders have learned something new. Probability is a fascinating subject that can help us understand the world better and make better decisions.
To master probability, 6th graders must practice and apply what they have learned to different situations. Here are some tips for further practice:
- Review the concepts and rules that you have learned in this article
- Try to solve some probability problems on your own or with a friend
- Play some games of chance and calculate your chances of winning
- Use simulations to explore different scenarios and outcomes
- Conduct some experiments and analyze the data
- Check out the web links that we have provided for more resources
Remember that learning probability is not only useful but also fun. So keep practicing and have fun!
