Algebraic expressions worksheets for 6th Graders: Learn and Practice
-
Eager to find an outstanding resource for your 6th graders to learn and practice variable expressions in algebra? Then you are in the right place. This article will introduce your students to the world of fun, mastering all algebraic expressions concepts of the 6th Grade math curriculum. Also, this article will provide an overview of Mathskills4kids’ algebraic expressions worksheets for 6th Graders that you can download and print for your homeschool or classroom use.
Solve algebraic expressions like a pro with our 6th Grade math worksheets.
Are you ready to take your 6th graders’ math skills to the next level? Do you want to help them learn how to solve variable expressions in algebra easily? If you answered yes, then you are in the right place! This article will show your learner how to solve algebraic expressions like a pro using our 6th-grade math worksheets.
In this article, your 6th graders will learn the basics of writing, evaluating, identifying, sorting, and solving variable expressions with different types of numbers and operations. They will also learn how to use the properties of multiplication to write equivalent expressions in different forms.
By the end of this article, your Grade 6 students will have a solid foundation of variable expressions that will help them succeed in algebra and beyond!
-
BROWSE THE WEBSITE
-
DOWNLOAD FREE WORKSHEETS
-
-
GRADE 6 MATH TOPICS
- Whole numbers
- Multiplication
- Division
- Exponents and square roots
- Number theory
- Decimals
- Add & subtract decimals
- Multiply & divide decimals
- Fractions & mixed numbers
- Add & subtract fractions
- Multiply fractions
- Divide fractions
- Integers
- Operations with integers
- Mixed operations
- Rational numbers
- Problems solving
- Ratio & proportions
- Percentages
- Measuring units
- Money math
- Consumer math
- Telling time
- Coordinate graph
- Algebraic expressions
- One step equations
- Solve & graph inequalities
- Two-step equations
- 2D Geometry
- Symmetry & transformation
- 3D Shapes
- Geometry measurement
- Data and Graphs
- Statistics
- Probability
-
-
Writing algebraic expressions with two variables
 Print it...
Print it...
-
Writing algebraic expressions from word problems
 Print it...
Print it...
-
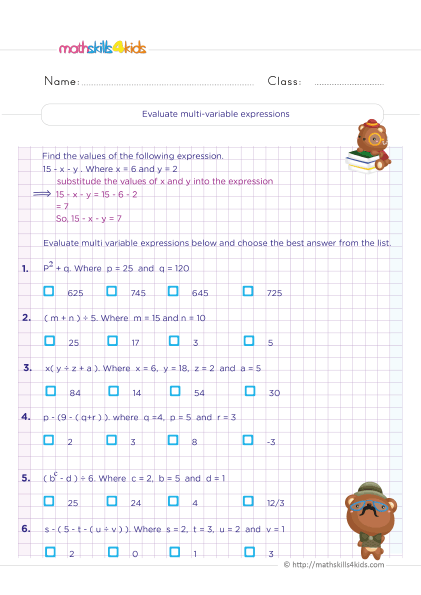
Evaluating algebraic expressions exercises with answers
 Print it...
Print it...
-
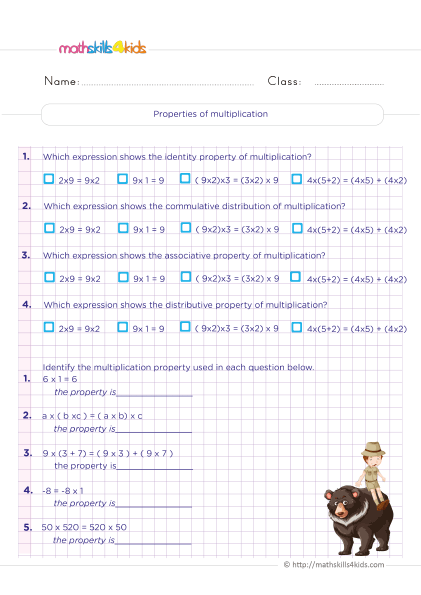
Commutative, associative and distributive properties of multiplication
 Print it...
Print it...
-
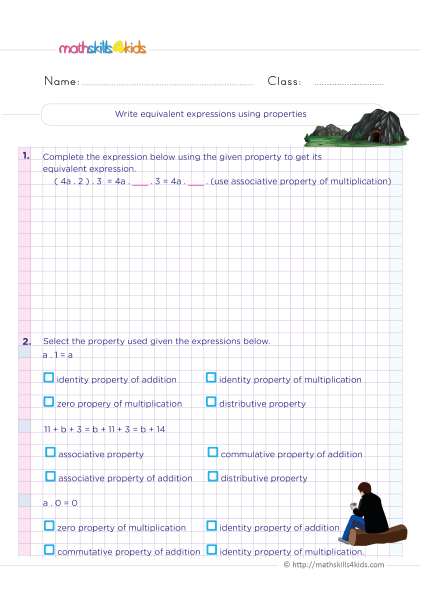
Writing equivalent expression using properties
 Print it...
Print it...
-
How to identify equivalent expressions practice
 Print it...
Print it...
-
Writing algebraic expressions with two variables
-
Buying is supporting us!
Buy Now...
-
-
What are variable expressions?
Variable expressions are mathematical phrases that contain numbers, variables, and operations. Variables are symbols, usually letters, that represent unknown or changing values. For example, x, y, and z are common variables.
Operations are symbols that indicate what to do with the numbers and variables, such as addition (+), subtraction (-), multiplication (×), and division (÷). For example, 3x + 5 is a variable expression that means "three times x plus five."
-
Why are variable expressions important for 6th Graders?
Variable expressions are important for 6th graders because they allow them to use algebra to model real-world situations and problems. For example, if we want to calculate the area of a rectangle with length x and width y, we can use the variable expression xy to represent the area.
If you know the x and y values, you can substitute them into the expression and find the area. For example, if x = 10 and y = 8, then xy = 10 × 8 = 80. This means that the area of the rectangle is 80 square units.
Variable expressions also help us to compare and analyze different situations and scenarios using algebra. For example, if you want to compare the costs of two different plans for a cell phone service, you can use variable expressions to represent the costs. For instance:
Plan A charges $20 monthly plus $0.10 per minute of talk time. Plan B charges $30 monthly plus $0.05 per minute of talk time. You can use the variable expression 20 + 0.1m to represent the cost of Plan A for m minutes of talk time. You can use the variable expression 30 + 0.05m to represent the cost of Plan B for m minutes of talk time. You can then use these expressions to compare the costs for different values of m and find out which plan is cheaper or more expensive.
-
How to master variable expressions in algebra using Mathskills4kids 6th-grade math worksheets
Now that your 6th graders know what variable expressions are and why they are important, let's see how they can master them using Mathskills4kids’ 6th-grade math worksheets.
Our worksheets cover the following topics:
- Write variable expressions up to two operations: Learn the basics of writing expressions with one or two operations
- Evaluate variable expressions with decimals, fractions, and mixed numbers: Practice substituting values and simplifying expressions with different types of numbers
- Identify terms and coefficients: Understand the meaning and role of terms and coefficients in variable expressions
- Sort factors of variable expressions: Use the distributive property to sort factors into groups
- Identify equivalent expressions: Recognize when two expressions have the same value for any variable
- Solve for a variable using properties of multiplication: Apply the inverse property and the commutative property to isolate a variable.
- Write equivalent expressions using properties: Use the associative property and the distributive property to rewrite expressions in different forms.
Each topic includes a brief explanation, examples, practice problems, and answers. You can download and print our worksheets for free and use them as often as needed. You can also check your answers online using our answer keys.
Let's take a look at each topic in more detail.
-
Write variable expressions up to two operations.
In this topic, students will learn how to write variable expressions with one or two operations using numbers, variables, and symbols. For example, they will learn how to write an expression that means "the sum of a number and seven" or "the product of five and a number minus two." They will also learn how to write expressions matching a word problem or situation.
To write a variable expression with one or two operations, follow these steps:
- Identify the unknown or changing value in the problem or situation and assign a variable to it.
- Identify the numbers and operations involved in the problem or situation.
- Write an expression using the variable, numbers, and symbols that match the problem or situation.
For example, suppose you want to write an expression that means "the sum of a number and seven." Here are the steps:
- Identify the unknown or changing value in the problem or situation and assign a variable to it. Let's use x to represent the number.
- Identify the numbers and operations involved in the problem or situation. The numbers are x and 7. The operation is addition (+).
- Write an expression using the variable, numbers, and symbols that match the problem or situation. The expression is x + 7.
Here are some more examples of writing variable expressions with one or two operations:
- The product of five and a number minus two: 5x - 2
- The quotient of a number and four plus three: x ÷ 4 + 3
- The difference of eight and a number: 8 - x
- The perimeter of a square with side length x: 4x
- The cost of m apples at $0.50 each: 0.5m
Notwithstanding, using Mathskills4kids.com worksheet, your 6th Grade students can practice writing variable expressions with one or two operations. They will find problems like these:
- Write an expression that means "the product of six and a number plus four."
- Write an expression that means "the quotient of a number and three minus five."
- Write an expression for the area of a rectangle with length x and width y.
- Write an expression for the cost of n books at $12 each.
- Write an expression that matches this situation: A car rental company charges $25 per day plus $0.15 per mile.
-
Evaluate variable expressions with decimals, fractions, and mixed numbers
In this topic, students will learn how to evaluate variable expressions with decimals, fractions, and mixed numbers. Evaluating means substituting a given value for the variable and simplifying the expression using the order of operations. For example, 6th graders will learn how to evaluate an expression like 3x + 5 when x = 2.5 or x = 3/4.
To evaluate a variable expression with decimals, fractions, or mixed numbers, follow these steps:
- Substitute the given value for the variable in the expression.
- Simplify the expression using the order of operations. Remember to follow these rules:
- Perform any calculations inside parentheses first.
- Perform any calculations involving exponents or roots next.
- Perform any calculations involving multiplication or division from left to right.
- Perform any calculations involving addition or subtraction from left to right.
- Write the final answer in simplest form.
For example, suppose you want to evaluate the expression 3x + 5 when x = 2.5. Here are the steps:
- Substitute the given value for the variable in the expression. Replace x with 2.5 in the expression: 3(2.5) + 5
- Simplify the expression using the order of operations. Perform any calculations involving multiplication or division from left to right: 7.5 + 5
- Perform any calculations involving addition or subtraction from left to right: 12.5
- Write the final answer in simplest form. The answer is 12.5.
Here are some more examples of evaluating variable expressions with decimals, fractions, or mixed numbers:
- Evaluate the expression x - 4 when x = 1.2: (1.2) - 4 = -2.8
- Evaluate the expression x ÷ 2 + 3 when x = 3/4: (3/4) ÷ 2 + 3 = (3/8) + 3 = (3/8) + (24/8) = (27/8)
- Evaluate the expression 2x + y when x = -0.5 and y = 1/2: 2(-0.5) + (1/2) = -1 + (1/2) = (-1/1) + (1/2) = (-2/2) + (1/2) = (-1/2)
- Evaluate the expression xy - z when x = 1.5, y = 2/3, and z = -0.25: (1.5)(2/3) - (-0.25) = (3/2)(2/3) - (-0.25) = (6/6) - (-0.25) = 1 - (-0.25) = 1 + 0.25 = 1.25
Also, your students can practice evaluating variable expressions with decimals, fractions, or mixed numbers using our worksheet. They will find problems like these:
- Evaluate the expression x + y when x = -0.75 and y = -1/4.
- Evaluate the expression x × y when x = 4/5 and y = -1.25.
- Evaluate the expression x ÷ y + z when x = -0.6, y = -3/5, and z = -0.4.
- Evaluate the expression xy - z when x = -1/3, y = -0.9, and z = -0.6.
- Evaluate the expression x² + y² when x =7/2, and y = 6.5
-
Identify terms and coefficients: Understand the meaning and role of terms and coefficients in variable expressions.
One of algebra's most important skills is identifying the terms and coefficients in a variable expression. A term is a number, a variable, or a product of numbers and variables. A coefficient is the numerical factor of a term that contains a variable.
For example, in the expression 3x + 5y - 2, there are three terms: 3x, 5y, and -2. The coefficients of the terms with variables are 3 and 5. The term -2 has no coefficient because it does not contain a variable.
To identify the terms and coefficients in a variable expression, we can follow these steps:
- Separate the expression into parts using the addition or subtraction signs as boundaries. Each part is a term.
- Look for the numerical factor of each term that contains a variable. That is the coefficient of that term.
- If a term does not have a numerical factor, then the coefficient is 1 or -1, depending on the sign of the term.
- If a term does not have a variable, it does not have a coefficient.
For example, identify the terms and coefficients in the expression 4x - y + 7.
- The expression has three terms: 4x, -y, and 7.
- The coefficient of 4x is 4.
- The coefficient of -y is -1.
- The term 7 does not have a coefficient.
Identifying terms and coefficients helps students to understand how different parts of an expression relate to each other. It also prepares them for more advanced topics in algebra, such as simplifying, combining, and factoring expressions.
-
Sort factors of variable expressions: Use the distributive property to sort factors into groups
Another useful skill in algebra is to sort factors of variable expressions into groups. This means to rewrite an expression as a product of two or more factors. For example, the expression x + 2 can be rewritten as (x + 2) x 1, which shows that it has two factors: x + 2 and 1.
We can use the distributive property to sort factors of variable expressions into groups. The distributive property states that a(b + c) = ab + ac. This means we can multiply a factor by a sum or difference of two terms by multiplying it by each term separately and then adding or subtracting the results. For example, 3(x + y) = 3x + 3y.
The distributive property also works in reverse. This means we can rewrite an expression as a product of two factors by finding a common factor in each term and then factoring it. For example, 6x + 9 = 3(2x + 3).
To sort factors of variable expressions into groups using the distributive property, you can follow these steps:
- Look for a common factor in each term of the expression. A common factor is a number or a variable that divides evenly into each term.
- Write the expression as a product of the common factor and another factor containing each term's remaining parts.
- Check your answer using the distributive property to expand your product and see if you return to the original expression.
Let's try an example: Sort factors of variable expressions into groups for the expression x2 + x - 6.
- The expression has three terms: x2, x, and -6.
- The only common factor in all three terms is 1, which does not help us to sort factors into groups.
- However, we can use another method to find two factors that multiply to give x2 + x - 6. We can look for two numbers that add up to 1 (the coefficient of x) and multiply by -6 (the constant term). These numbers are 3 and -2.
- We can rewrite x2 + x - 6 as (x + 3)(x - 2), which shows that it has two factors: x + 3 and x - 2.
- We can check our answer using the distributive property to expand our product: (x + 3)(x - 2) = x2 - 2x + 3x - 6 = x2 + x - 6.
Sorting factors of variable expressions into groups helps learners to simplify and compare expressions. It also prepares them for more advanced topics in algebra, such as solving equations and inequalities.
-
Identify equivalent expressions: Recognize when two expressions have the same value for any variable.
Another vital skill in algebra is to identify equivalent expressions. Equivalent expressions are expressions that have the same value for any value of the variable. For example, the expressions x + y and y + x are equivalent because they have the same value for any x and y values.
To identify equivalent expressions, we can use different methods, such as:
- Simplifying expressions by using the properties of operations, such as the commutative, associative, and distributive properties.
- Substituting values for the variable and comparing the results.
- Using logical reasoning and algebraic rules.
Let's try an example: Identify whether the expressions 2x + 3 and x + x + 3 are equivalent.
- One method is to simplify the expressions by using the properties of operations. We can rewrite x + x as 2x by using the definition of multiplication. Then we can see that 2x + 3 and 2x + 3 are the same expressions, so they are equivalent.
- Another method is to substitute values for x and compare the results. For example, if we let x = 5, then 2x + 3 = 2 x 5 + 3 = 13 and x + x + 3 = 5 + 5 + 3 = 13. We can see that both expressions have the same value for x = 5.
We can try other values of x and see that they always have the same value, so they are equivalent.
- A third method is to use logical reasoning and algebraic rules. We can see that adding x to itself is the same as multiplying x by 2, so x + x = 2x. Then we can see that adding 3 to both sides of an equation does not change its solution, so x + x + 3 = 2x + 3.
Therefore, the expressions are equivalent.
Identifying equivalent expressions helps 6 Grade students to simplify and manipulate expressions. It also prepares them for more advanced topics in algebra, such as solving equations and inequalities.
-
Solve for a variable using properties of multiplication: Apply the inverse property and the commutative property to isolate a variable.
Another essential skill in algebra is to solve for a variable using properties of multiplication. This means finding a variable's value that makes an equation true. For example, in the equation 2x = 10, we want to find the value of x that makes both sides equal.
To solve for a variable using properties of multiplication, you can use two main properties: the inverse property and the commutative property.
- The inverse property states that multiplying by a number's reciprocal (or multiplicative inverse) is the same as dividing by that number. For example, multiplying by 1/2 is the same as dividing by 2.
- The commutative property states that changing the order of factors does not change their product. For example, 2 x x = x x 2.
To solve for a variable using properties of multiplication, we can follow these steps:
- Identify the coefficient of the variable in the equation. The coefficient is the number that is multiplied by the variable.
- Multiply both sides of the equation by the reciprocal of the coefficient. This will cancel out the coefficient on one side and leave only the variable.
- Use the commutative property to write the variable on one side and the solution on the other.
Let's try an example: Solve for x in the equation 3x = -12.
- The coefficient of x in the equation is 3.
- We multiply both sides of the equation by the reciprocal of 3, which is 1/3.
This gives us (1/3) x (3x) = (1/3) x (-12).
- We simplify both sides by using the inverse property. This gives us x = -4.
- We use the commutative property to write x on one side and -4 on the other. This gives us x = -4.
Solving for a variable using properties of multiplication helps 6th graders to find unknown values in equations. It also prepares them for more advanced topics in algebra, such as solving equations with fractions and decimals.
-
Write equivalent expressions using properties: Use the associative and distributive properties to rewrite expressions in different forms.
One of the most valuable skills in algebra is being able to rewrite expressions in different forms. This can help you simplify, compare, and solve expressions more easily. There are two main properties that you can use to do this: the associative property and the distributive property.
The associative property says that we can change the grouping of terms or factors in an expression without changing its value. For example, (a + b) + c = a + (b + c) and (a x b) x c = a x (b x c). This property works for addition and multiplication but not for subtraction and division.
The distributive property says that we can multiply a factor by a sum or difference by multiplying each term inside the parentheses by the factor outside. For example, a x (b + c) = a x b + a x c and a x (b - c) = a x b - a x c. This property can help us expand or factor expressions.
Let's see some examples of how to use these properties to write equivalent expressions.
Example 1: Write an equivalent expression for 3 x (4 + 5) using the distributive property.
Solution: Using the distributive property, we can multiply 3 by each term inside the parentheses.
3 x (4 + 5) = 3 x 4 + 3 x 5
= 12 + 15
= 27
The expression 3 x (4 + 5) is equivalent to 27.
Example 2: Write an equivalent expression for (2 x y) x (3 x z) using the associative property.
Solution: We can use the associative property to change the grouping of factors in the expression.
(2 x y) x (3 x z) = 2 x (y x 3) x z
= 2 x (3 x y) x z
= 6 x y x z
The expression (2 x y) x (3 x z) is equivalent to 6 x y x z.
Bonus: Engaging resources to enhance algebraic expression skills in 6th-grade
If you want to enhance your 6th graders' variable expression skills, check out these engaging online resources for more practice:
- Math Games: Algebraic Expressions (https://www.mathgames.com/skill/6.1-algebraic-expressions). This website offers interactive games and quizzes that test students’ knowledge of algebraic expressions. They can choose from different difficulty levels and topics, such as simplifying, evaluating, and writing expressions.
- Khan Academy: Introduction to Algebra (https://www.khanacademy.org/math/cc-sixth-grade-math/cc-6th-expressions-and-variables). This website provides video lessons and exercises covering algebra basics, such as variables, expressions, equations, and inequalities. 6th Grade students can watch videos at their own pace and track their progress with badges and points.
- Math Playground: Algebra Puzzles (https://www.mathplayground.com/index_prealgebra.html). This website features fun and challenging puzzles that require students to use logic and algebra skills to find missing numbers or variables. They can drag and drop numbers and symbols to create equations and solve for unknowns.
-
-
Thank you for sharing the links of MathSkills4Kids.com with your loved ones. Your choice is greatly appreciated.
Conclusion
By practicing these skills, your learner can solve algebraic expressions like a pro with Mathskills4kids’ 6th-grade math worksheets.
Also, check out these engaging resources to improve your 6th graders’ algebraic expression skills:
- Math Games: Playing fun and interactive games helps students practice writing and evaluating variable expressions.
- Math Videos: Watch short and clear videos that explain how to use properties and terms in variable expressions.
- Math Worksheets: Download and print free worksheets that give students plenty of practice with variable expressions.
We hope you enjoyed this article and learned something new. Remember, algebra is easy if your students know how to use variable expressions.
Please encourage them to keep practicing and have fun with math!
